
前回の内容はこちらです.
問題がほとんど同じですが,使っていい文字が異なります.
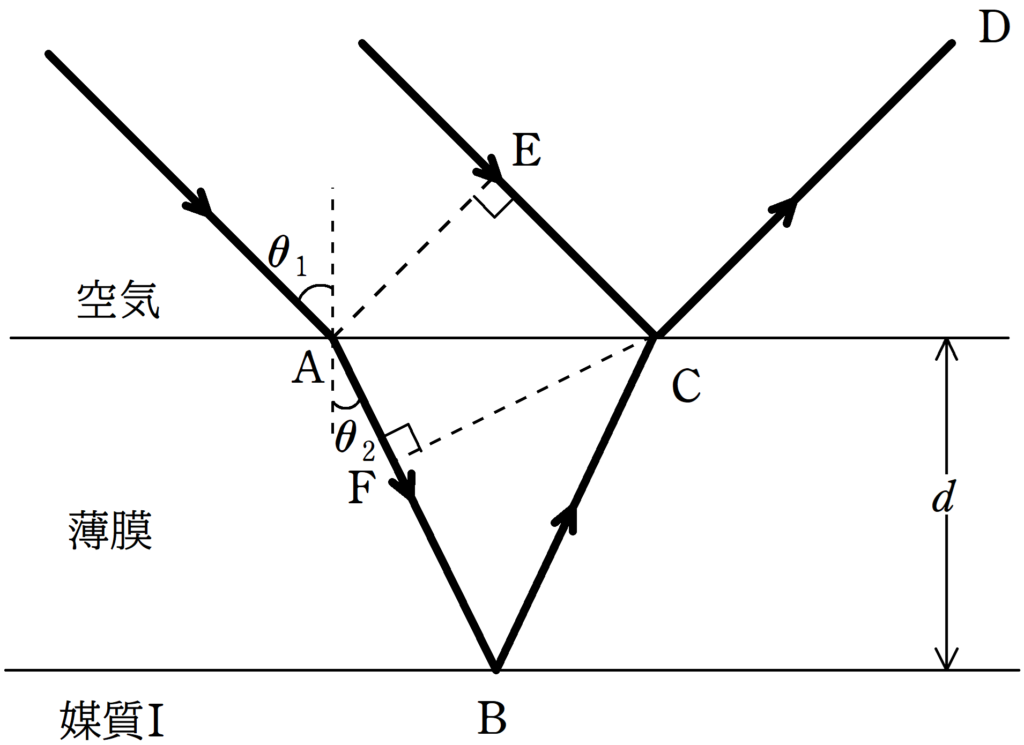
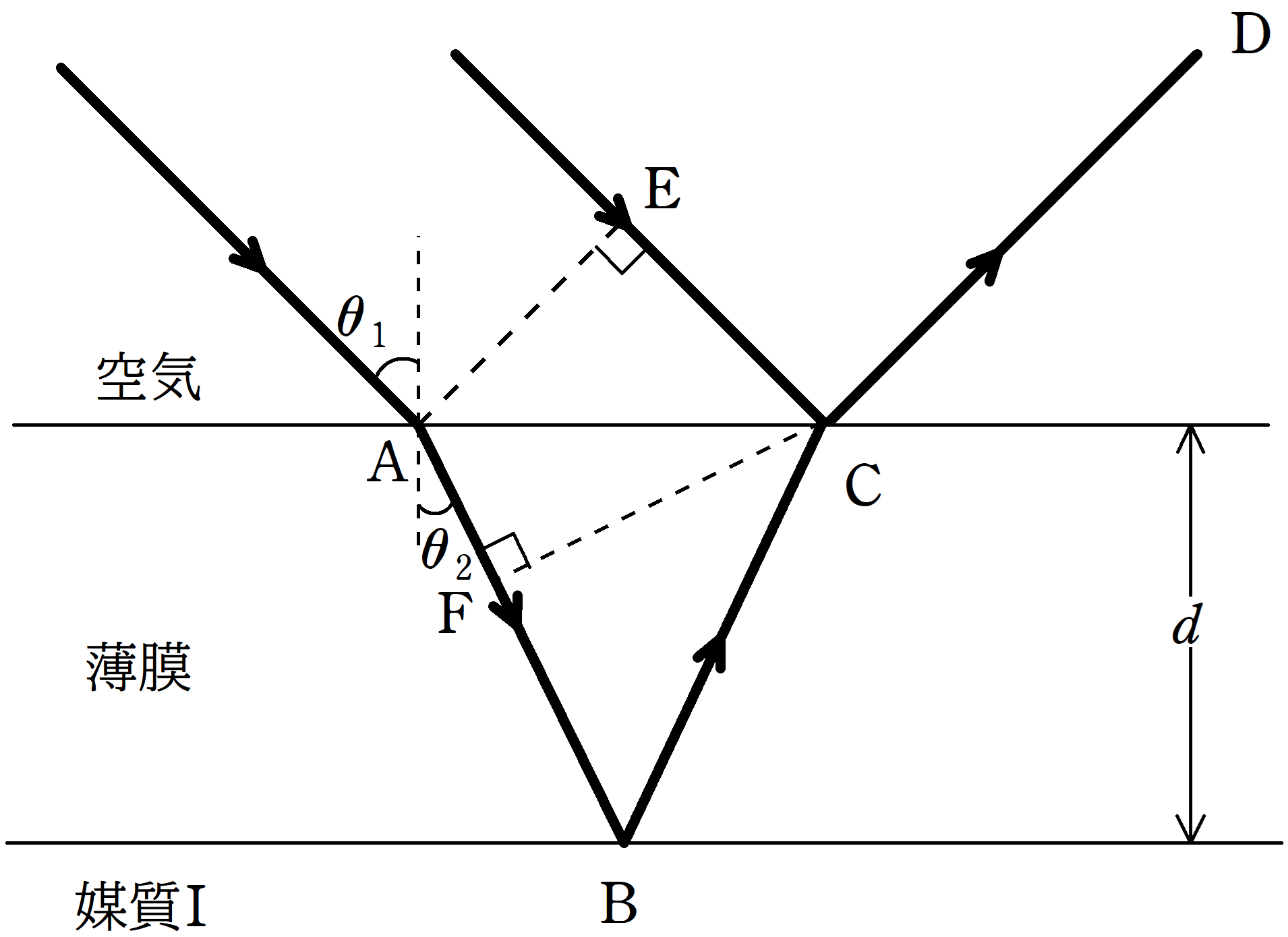
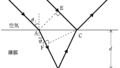
光を薄膜に入射し,反射光の干渉を考える.図のA,Eで位相がそろった波について,Aを通る光は,F,を通過し,媒質Iとの境界であるBで反射し,Cから空気中へ出てDへ行く.一方,Eを通る光はCで反射し,Dに向かい2つの経路の光が干渉する.空気に対する薄膜の屈折率を$n(>1)$とし,A,Cにおける入射角を$\theta_{1}$,Aにおける屈折角を$\theta_{2}$とする.また,薄膜の厚さを$d$であり,空気中の光の波長を$\lambda$とする.このとき,次の問いに答えよ.
(1) 媒質Iが空気のとき,2つの光の強め合いの条件と弱め合いの条件をそれぞれ,$d$,$\theta_{1}$,$\lambda$,$n$,自然数$m$を用いて表せ.
(2) 媒質Iの空気に対する相対屈折率が$n^{\prime}(>n)$のとき,2つの光の強め合いの条件と弱め合いの条件をそれぞれ,$d$,$\theta_{1}$,$\lambda$,$n$,自然数$m$を用いて表せ.
<解答>
(1)

位相差は次のことをチェックしましょう.
地点Pにおける2つの波の位相差を$\phi$,整数を$m$とすると,
強め合いの条件:$\phi=2\pi m$
弱め合いの条件:$\phi=(2m+1)\pi $
位相差$\phi$は次の3つの要素で決まる.
$\phi_{1}$:初期位相のずれ
同位相なら$\phi_{1}=0$,逆位相なら$\phi_{1}=\pi$
$\phi_{2}$:反射による位相のずれ
自由端反射なら$\phi_{2}=0$,固定端反射なら$\phi_{2}=\pi$
$\phi_{3}$:距離の差(屈折率が変わることで生じる光学的な距離の差を含む)による位相のずれ
距離の差が$\Delta l$のとき,次の比例式を立てて,位相差$\phi_{3}$を計算する.
$2\pi : \lambda =\phi_{3} : \Delta l$
$\therefore \phi_{3}=\dfrac{2\pi}{\lambda}\Delta l$
このとき,位相差$\phi$は
$\phi=\phi_{1}+\phi_{2}+\phi_{3}$

また,固定端反射なのか自由端反射なのかは次の図で確認していきましょう.
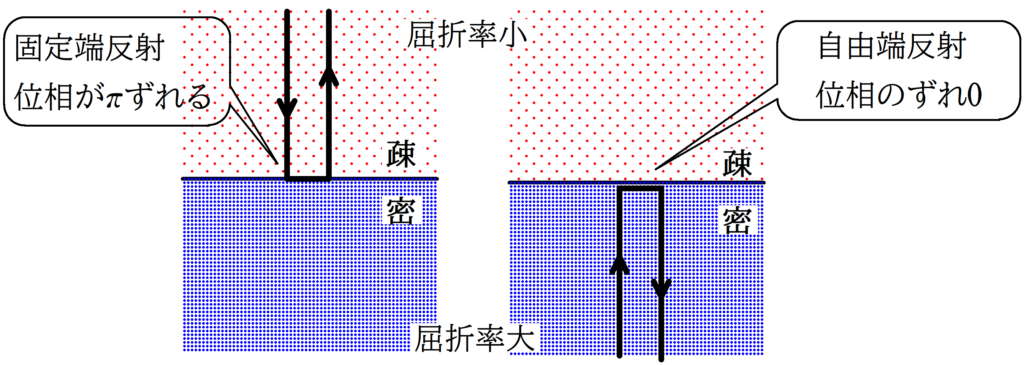

また,CとFは同位相なんだよね.理由はこちらで説明しています.
なので,経路による位相差はFBC部分だけ考えればいいんだね.
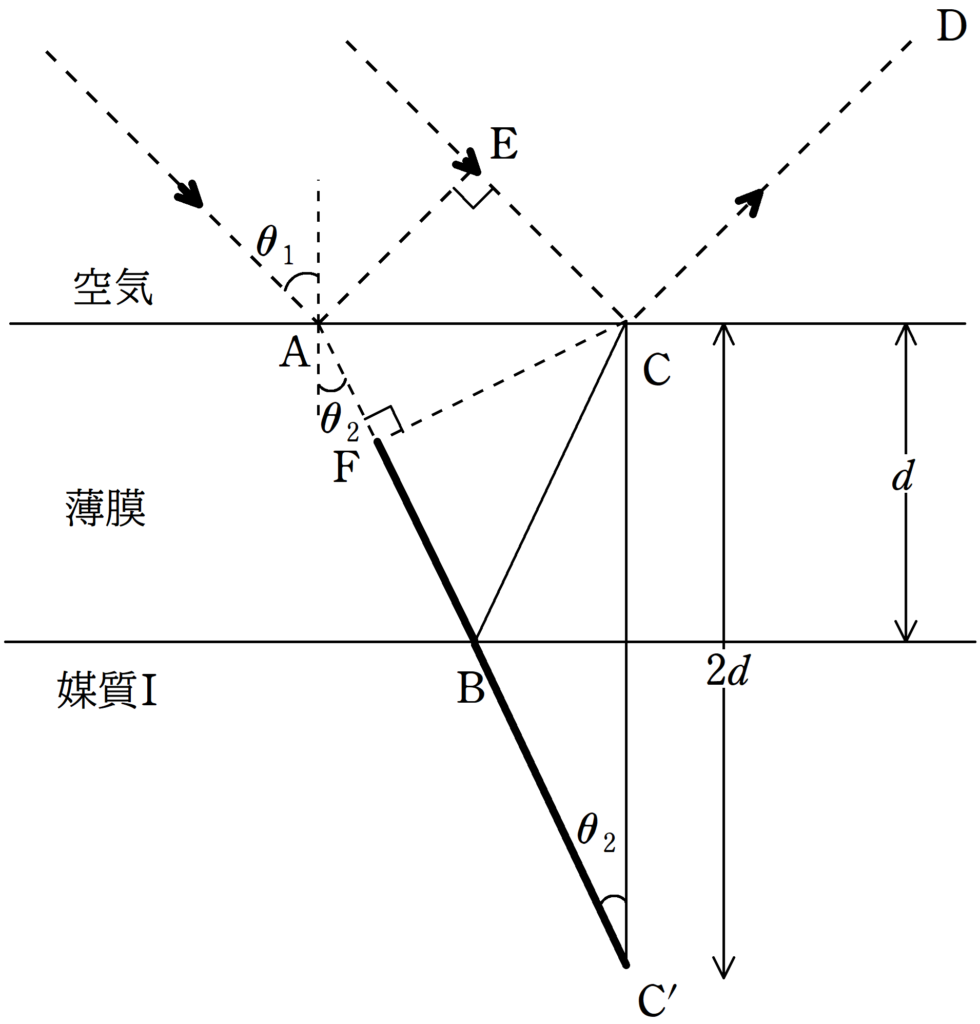

FBCの長さは次のように計算するとよいでしょう.
BCを媒質Iと薄膜の境界線に対して線対称移動したときのCに対応する点を$\rm C^{\prime}$とします.
このとき,$\rm FB+BC=FB+BC^{\prime}$であり,直角三角形$\rm CC^{\prime}F$に着目すると$\rm FC^{\prime}$の長さは
${\rm FC^{\prime}}=2d\cos\theta_{2}$
となります.
また,Cでは固定端反射,Bでは自由端反射するので,反射による位相差は$\pi$です.
したがって,位相差$\varDelta \varphi$は次のようになります.
$\varDelta \varphi=\dfrac{2\pi}{\dfrac{\lambda}{n}}\cdot 2d\cos\theta_{2}+\pi$ $\dots (\ast)$

しかし,今回は$\theta_{2}$を使えないので,屈折の法則で$\theta_{1}$との関係式をつくりましょう.
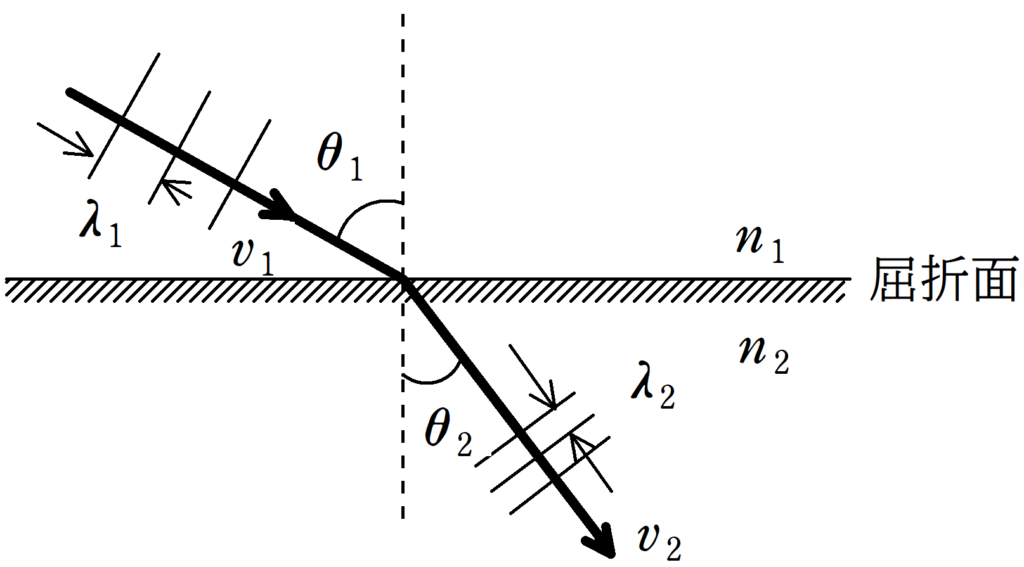
速さ$v_{1}$,波長$\lambda_{1}$の波が入射角$\theta_{1}$で屈折面に入射し,その後屈折角が$\theta_{2}$,速さ$v_{2}$,波長が$\lambda_{2}$となった.入射側の媒質の屈折率を$n_{1}$,屈折する側の媒質の屈折率を$n_{2}$とすると,次の関係が成り立つ.
$\dfrac{v_{1}}{v_{2}}=\dfrac{\sin\theta_{1}}{\sin\theta_{2}}$
また,屈折により,媒質の振動数$f$および周期$T$は変化しないことから,次のことが成り立つ.
$\dfrac{v_{1}}{v_{2}}=\dfrac{\cancel{f}\lambda_{1}}{\cancel{f}\lambda_{2}}=\dfrac{\lambda_{1}}{\lambda_{2}}$
一方,屈折率との関係は次のようになる.
$n_{1}\sin\theta_{1}=n_{2}\sin\theta_{2}$
$n_{1}v_{1}=n_{2}v_{2}$
$n_{1}\lambda_{1}=n_{2}\lambda_{2}$
最後に,$n_{12}=\dfrac{n_{2}}{n_{1}}$を屈折率$n_{1}$の媒質に対する屈折率$n_{2}$の媒質の相対屈折率という.
$1\cdot \sin\theta_{1}=n\sin\theta_{2}$
$\therefore \cos\theta_{2}=\dfrac{\sqrt{n^{2}-\sin^{2}\theta_{1}}}{n}$ $\dots (2\ast)$
$(2\ast)$を$(\ast)$に代入すると
$\varDelta \varphi=\dfrac{2\pi}{\dfrac{\lambda}{n}}\cdot 2d\cdot \dfrac{\sqrt{n^{2}-\sin^{2}\theta_{1}}}{n}+\pi$
強め合いの条件は
$\dfrac{2\pi}{\dfrac{\lambda}{n}}\cdot2d\cdot \dfrac{\sqrt{n^{2}-\sin^{2}\theta_{1}}}{n}+\pi=2\pi m$
$\therefore \dfrac{4\pi d\sqrt{n^{2}-\sin^{2}\theta_{1}}}{\lambda}=(2m-1)\pi$ (答)
弱め合いの条件は
$\dfrac{2\pi}{\dfrac{\lambda}{n}}\cdot 2d\cdot \dfrac{\sqrt{n^{2}-\sin^{2}\theta_{1}}}{n}+\pi=(2m+1)\pi$
$\therefore \dfrac{4\pi d\sqrt{n^{2}-\sin^{2}\theta_{1}}}{\lambda}=2m\pi$ (答)

使える文字が異なると,結構違う形になるんだね.
(2)

Cでは固定端反射,Bでも固定端反射するので,反射に位相差は0となります.
強め合いの条件は
$\dfrac{2\pi}{\dfrac{\lambda}{n}}\cdot 2d\cdot \dfrac{\sqrt{n^{2}-\sin^{2}\theta_{1}}}{n}=2\pi m$
$\therefore \dfrac{4\pi d\sqrt{n^{2}-\sin^{2}\theta_{1}}}{\lambda}=2\pi m$ (答)
弱め合いの条件は
$\dfrac{2\pi}{\dfrac{\lambda}{n}}\cdot 2d\cdot \dfrac{\sqrt{n^{2}-\sin^{2}\theta_{1}}}{n}=(2m-1)\pi$
$\therefore \dfrac{4\pi d\sqrt{n^{2}-\sin^{2}\theta_{1}}}{\lambda}=(2m-1)\pi$ (答)

次回の内容はこちら.
コメント
[…] https://physicmath.net/6075/,6075,physicmath.net投稿,publish 分野別波動演習問題演習問題(標準)高校物理 シェアする Twitter Facebook はてブ LINE physicmathをフォローする physicmath Physicmath(フィジクマス) […]
[…] […]